Introduction:
Hello! I am Hugo Vanneuville, I am a researcher in math. Since January 2021, I am a CNRS researcher at
Institut Fourier (Université Grenoble Alpes) in the
probability team. Before this, I have completed a PhD at
Institut Camille Jordan (Université Lyon 1) under the direction of
Christophe Garban and I have been a post-doctoral resarcher at the
math department of ETH Zürich under the direction of
Vincent Tassion.
I am mainly interested in the study of phase transition and noise sensitivity phenomenons in percolation models. You can find more information below.
2 years postdoctoral position in Probability:
Applications are invited for a 2 years post-doc position in Probability at Institut Fourier (Grenoble) starting in September 2026. The deadline for applications is March 23, 2026. Interviews will be held during the week of April 6, and responses will be provided nol ater than the week of April 13.
The postdoctoral position is funded by the ANR "Agence Nationale de la Recherche" project COCONUT "Correlations in percolation, chaos and noise sensitivity".
The research areas include: Percolation, First Passage Percolation, Ising model, disordered chaos, noise sensitivity, sharpness of phase transitions, planar statistical mechanics models. Depending on the subject, we will propose a co-supervision with Barbara Dembin (Strasbourg), or other members of the ANR project.
There will be several workshops organized every year for this ANR project. Members of the project (in addition to Barbara Dembin and myself) include Loren Coquille (Grenoble), Malo Hillairet (Grenoble), Franco Severo (Paris), Jean Vereecke (Grenoble) and Julien Verges (Stockholm).
The salary is 2 676 euros per month (between year 0 and 3 after PhD) or 3 006 euros per month (between year 3 and 7 after PhD) (net amounts before withholding tax). The postdoc would obtain financial support to attend conferences and invite collaborators.
The starting date is September 2026 but a slightly later date is possible if necessary.
In order to apply to this position, send a CV and a three or four pages description of research interests (report on the work carried out and brief presentation of topics that interest you) to both barbara.dembin [ at ] math.unistra [dot] fr and hugo.vanneuville [at] univ-grenoble-alpes [dot] fr. Applicants should also arrange for up to three letters of recommendation (addressed by the authors directly to the same emails). All documents can be written either in English or in French.
Research interests:
I am interested in phase transition and noise sensitivity phenomenons in percolation models and in the universality properties of these models (in 2D in most of my works). In percolation theory, one studies the connectivity properties of random sets (such as a random subset of edges of a given lattice, or level sets of a random function). When the density of the random set reaches some critical value, fractal structures appear; they are the sign of the emergence of interactions at all scales.
More specifically, the following three questions are guiding my research (among others):
Can we find new ways of formalising the fact that a threshold effect appears if the event that we study "depends little on every given coordinate"?
How can we show noise sensitivity properties without using any spectral tool?
Can we prove universality properties by using noise sensitivity?
(See also
here for an interview done by INSMI when I was recruited, in which I describe my field of research - in French).
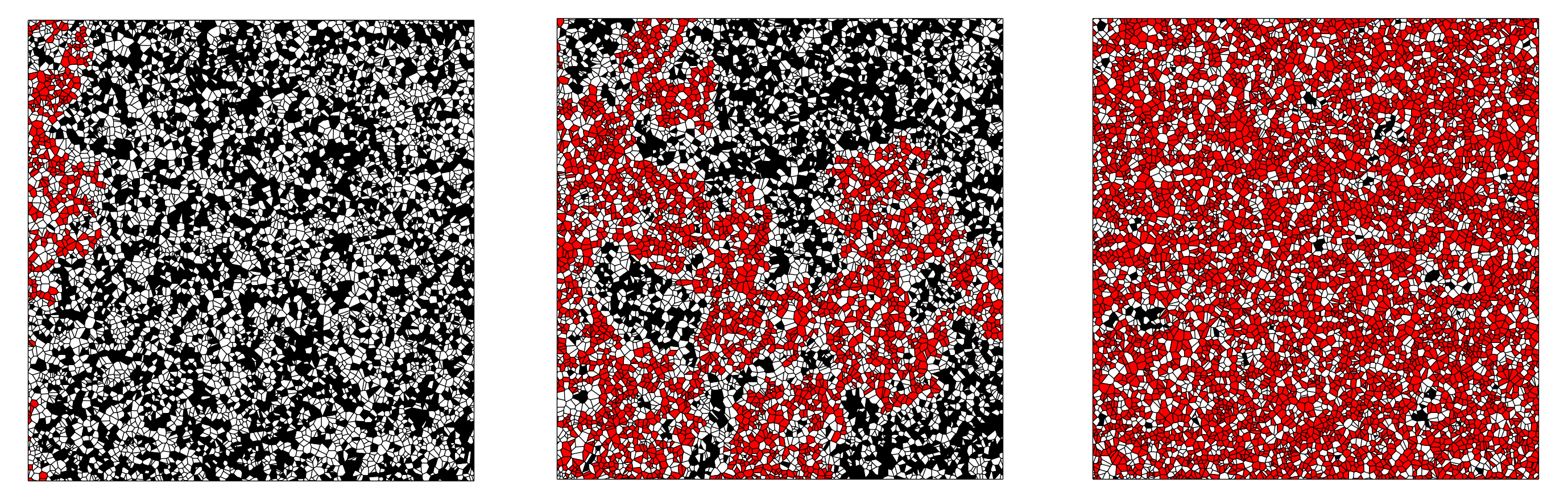
Figures: Percolation on a random tiling: each face is colored black with probability p=0.4, 0.5 and 0.6 respectively. In red: the points connected to the left side by a black path. There is a transition at p=0.5.
Research papers:
New approaches to noise sensitivity:
Noise sensitivity of crossings for high temperature Ising model
with Vincent Tassion
(Peprint)
Noise sensitivity of percolation via differential inequalities
with Vincent Tassion
(Proceedings of the London Mathematical Society, 2023)
About the phase transition in Bernoulli percolation:
Exponential decay of the volume for Bernoulli percolation: a proof via stochastic comparison
(Annales Henri Lebesgue, 2025)
Random nodal lines:
Existence of an unbounded nodal hypersurface for smooth Gaussian fields in dimension d >= 3
with Hugo Duminil-Copin, Alejandro Rivera and Pierre-François Rodriguez
(Annals of Probability, 2023 ; the published version contains one error, see the erratum. This error has been corrected in the arXiv version.)
The phase transition for planar Gaussian percolation models without FKG
with Stephen Muirhead and Alejandro Rivera, and with an appendix written by Laurin Köhler-Schindler
(Annals of Probability, 2023)
Bargmann-Fock percolation is noise sensitive
with Christophe Garban
(Electronic Journal of Probability, 2020)
The sharp phase transition for level set percolation of smooth planar Gaussian fields
with Stephen Muirhead
(Annales de l'Institut Henri Poincaré, prob. et stat., 2020)
The critical threshold for Bargmann-Fock percolation
with Alejandro Rivera
(Annales Henri Lebesgue, 2020)
Quasi-independence for nodal lines
with Alejandro Rivera
(Annales de l'Institut Henri Poincaré, prob. et stat., 2019)
Voronoi percolation, noise sensitivity and dynamics:
The annealed spectral sample of Voronoi percolation
(Annals of Probability, 2021)
Quantitative quenched Voronoi percolation and applications
(Annales de l'Institut Fourier, 2025 (but it is not a recent paper: on arXiv in 2018))
Annealed scaling relations for Voronoi percolation
(Electronic Journal of Probability, 2019)
Exceptional times for percolation under exclusion dynamics
with Christophe Garban
(Annales scientifiques de l'École Normale Supérieure, 2019)
Other writings (not for publication):
Sharpness of Bernoulli percolation via couplings
Overview and concentration results for nodal lines
(introductory text for the reading group on random nodal lines organized at Zürich in 2019)
Percolation in the plane: dynamics, random tilings and nodal lines
(PhD thesis under the direction of Christophe Garban -- the introduction is written in French and the chapters are written in English)
Slides
Small erratum
List of my co-authors:
Hugo Duminil-Copin,
Christophe Garban, Laurin Köhler-Schindler,
Stephen Muirhead, Alejandro Rivera,
Pierre-François Rodriguez,
Vincent Tassion
Teaching:
I taught at Université Lyon 1 during my PhD thesis. At Université Grenoble Alpes, I taught the course "Random models on lattices" with
Loren Coquille during the first semester of the year 2023-2024. You can find the course summary
here. During the second semester of the years 2021-2022, 2022-2023 and 2023-2024, I taught with Agnès Coquio the M1 course "Markov processes". During the first semester of the year 2025-2026, I teach (with
Charline Smadi) the exercices sessions for the M1 Probability course. For all these courses, do not hesitate to send me an e-mail if you want lecture notes.
Diffusion (in French):
J'ai fait un exposé lors du "Barcamp" organisé par les bibliothécaires de Lyon 1 en mai 2018. Vous pouvez trouver la vidéo
ici.
